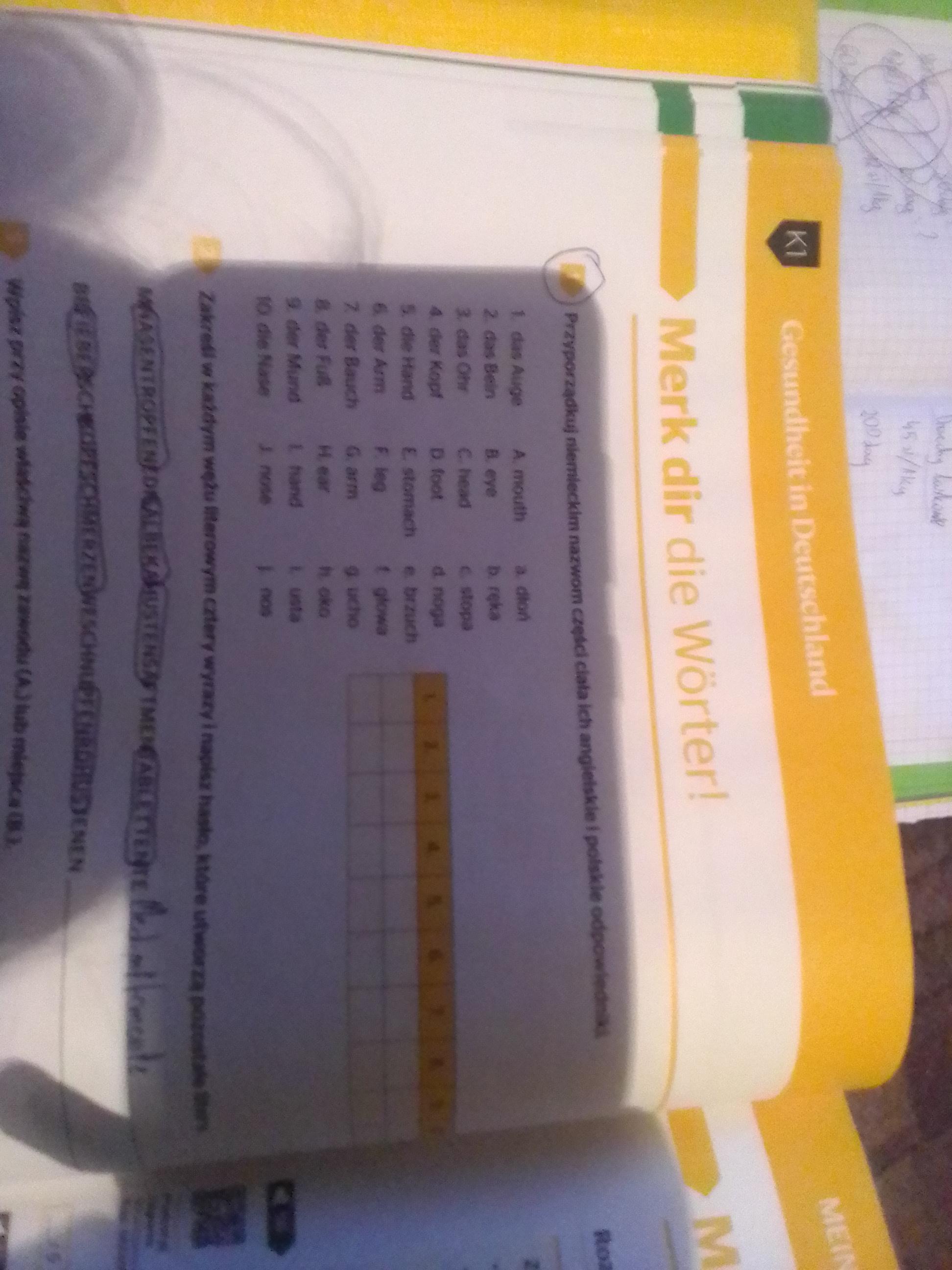
Look at the photo in the blog post and answer the questions. 1 What is the boy doing? Who do you think he's talking to? 2 Do you prefer calling or texting your friends and family? Why?​

question img

Odpowiedzi 1

Odpowiedź:

1.he's chaking massanges on his phone.i don't now.

2.nato musisz odpwiedzieć sam/sama tłumaczenie: Wolisz dzwonić lub wysyłać SMS-y do znajomych i rodziny? Dlaczego?​

Znasz odpowiedź? Dodaj ją tutaj!

Can't find the answer?

Other related questions that may help you

b Wykonaj dzielenie a) 3 1 7 6 4 8 8 1 8 2 8 8 9 d c 1 7 0 14:47 5 17 16:14 e 4 1 5 9 1199 7 6 2 2 0 2 0 6
question img

Odpowiedź:

a)3976        

 317648:8

-24

  =77

   -72

    =56

     -56

      ==

b)           2  32

            18288:9

            -18

             ==

              28

              -27

               =18

                -18

c)  3694

  51716:14

 -42

  =97

   -84

    131    

   -126

        56

       -56

d)        362

      17 014:47

     -141

      =291

      -282

       ==94

          -94

e)     370

   76220:206

  -618

   1442

  -1442

f)      2  9

    41591:199

   -398

      1791

     -1791

 

   

Szczegółowe wyjaśnienie: liczę na najlepszą;)

Przyporządkuj niemiecki nazwom części ciała ich angielskie i polskie odpowiedniki szybko daje 20 punktów zadanie 1 1. Das auge 2.Das bein 3.das ohr 4. Der kopf 5. Die hand 6. Der arm 7 der bauch 8. Der fub 9.der mund 10.die Nase A mouth a dłoń B eye b ręka C Head c stopa D foot d noga E stomach e brzuch F leg f głowa G arm g ucho H ear h oko I hand i usta J nose j nos
question img

1. B, h

2. F, d

3. H, g

4. C, f

5. I, a

6. G, b

7. E, e

8. D, c

9. A, i

10. J, j

Oblicz 2 do potęgi 7 podzielić przez 2 2 do potęgi 6 minus 4 do potęgi trzeciej 10 do potęgi 8 podzielić na 10 i 0,5 plus 3,7 do potęgi 0 4 i do potęgi trzeciej minus 3 do potęgi trzeciej 10 do potęgi 6 podzielić na 50 do potęgi drugiej 0,000 4​
question img

Odpowiedź i szczegółowe wyjaśnienie:

[tex]a)\ 2^7:2 = 2^7:2^1=2^{7-1}=2^6\\\\b)\ 10^8:1000000=10^8:10^6=10^{8-6}=10^2\\\\c)\ 4^3-3^3=64-27=37\\\\d)\ \dfrac{0,0004}{0,1^4}=\dfrac{4\cdot0,0001}{0,1^4}=\dfrac{4\cdot0,01^4}{0,1^4}=4\\\\e)\ 2^6-4^3=2^6-(2^2)^3=2^6=2^6=0\\\\f)\ (0,5+3,7)^0=1\\\\g)\ 10^6:50^2=10^6:(5\cdot10)^2=10^6:(25\cdot10^2)=10^6:25\cdot10^2=10^{6-2}:25=\\=10^4:25 = 10000:25 = 400[/tex]

[tex]h)\ 0,2^4:0,01^2=\dfrac{(2\cdot0,1)^4}{0,01^2}=\dfrac{2^4\cdot0,1^4}{((0,1)^2)^2}=\dfrac{2^4\cdot0,1^4}{0,1^4}=2^4[/tex]

Wykorzystano podstawowe własności potęgowania postaci:

[tex]a^0 = 1\\a^n:a^m = a^{n-m}\\\\(a^n)^m = a^{n\cdot m}\\\\(a\cdot b)^n = a^n \cdot b^n[/tex]

Oblicz sinx cosx sin2x cos2x tg2x jezeli tgx=2
question img

Odpowiedź: w załączniku.

Szczegółowe wyjaśnienie:

answer img

Other Język angielski Questions

Zaloguj się z Google

 lub

Zapomniałeś(aś) hasła?

Nie mam jeszcze konta, ale chcę je założyć Zarejestruj się

Wybierz język i region

English

United States

Polski

Polska

Português

Brasil

English

India

English

Philippines

Español

España

Bahasa Indonesia

Indonesia

Türkçe

Türkiye
How much to ban the user?
1 hour 1 day 100 years