Przedstaw w postaci potęgi liczby 3
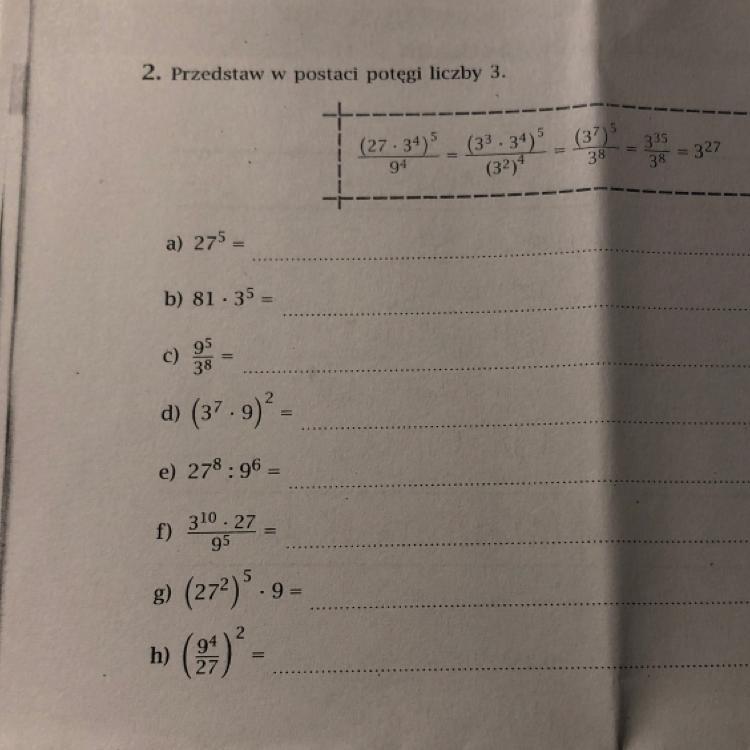
-
Temat:
Matematyka -
Autor:
buck -
Utworzono:
1 rok temu
Odpowiedzi 1
Odpowiedź:
[tex]\huge\boxed{zad.2}[/tex]
[tex]\huge\boxed{a)~~27^{5}=3^{15}}[/tex] [tex]\huge\boxed{b)~~81\cdot 3^{5}=3^{9}}[/tex]
[tex]\huge\boxed{c)~~\dfrac{9^{5}}{3^{8}} =3^{2}}[/tex] [tex]\huge\boxed{d)~~(3^{7} \cdot 9)^{2}=3^{18}}[/tex]
[tex]\huge\boxed{e)~~27^{8}\div 9^{6}=3^{12}}[/tex] [tex]\huge\boxed{f)~~\dfrac{3^{10}\cdot 27}{9^{5}} =3^{3}}[/tex]
[tex]\huge\boxed{g)~~(27^{2})^{5} \cdot 9= 3^{32}}[/tex] [tex]\huge\boxed{h)~~\left( \dfrac{9^{4}}{27} \right)^{2}=3^{10}}[/tex]
Szczegółowe wyjaśnienie:
Korzystamy ze wzorów:
- [tex]x^{n} \cdot x^{m} =x^{n+m}[/tex]
- [tex]x^{n} \div x^{m}=\dfrac{x^{n}}{x^{m}} =x^{n-m},~~zal.~~x\neq 0[/tex]
- [tex](x^{n})^{m} =x^{n\cdot m }[/tex]
- [tex]x^{n} \cdot y^{n} =(x\cdot y)^{n}[/tex]
- [tex]x^{n} \div y^{n}=\dfrac{x^{n}}{y^{n}} =\left( \dfrac{x}{y} \right)^{n} ,~~zal.~~y\neq 0[/tex]
[tex]a)~~27^{5}=(3^{3})^{5}=3^{3\cdot 5} = \huge\boxed{3^{15}}[/tex]
[tex]b)~~81\cdot 3^{5}=3^{4}\cdot 3^{5}=3^{4+5}=\huge\boxed{3^{9} }[/tex]
[tex]c)~~\dfrac{9^{5}}{3^{8}} =\dfrac{(3^{2})^{5}}{3^{8}} =\dfrac{3^{2\cdot 5}}{3^{8}} =\dfrac{3^{10}}{3^{8}} =3^{10-8}=\huge\boxed{3^{2}}[/tex]
[tex]d)~~(3^{7} \cdot 9)^{2}=(3^{7} \cdot 3^{2})^{2}=(3^{7+2} )^{2}=(3^{9} )^{2}=3^{9\cdot 2}=\huge\boxed{3^{18}}[/tex]
[tex]e)~~27^{8}\div 9^{6}=(3^{3})^{8}\div (3^{2})^{6}=3^{3\cdot 8}\div 3^{2\cdot 6}=3^{24}\div 3^{12}=3^{24-12}=\huge\boxed{3^{12}}[/tex]
[tex]f)~~\dfrac{3^{10}\cdot 27}{9^{5}} =\dfrac{3^{10}\cdot 3^{3}}{(3^{2})^{5}} =\dfrac{3^{10+3}}{3^{2\cdot 5}} =\dfrac{3^{13}}{3^{10}} =3^{13-10}=\huge\boxed{3^{3}}[/tex]
[tex]g)~~(27^{2})^{5} \cdot 9=((3^{3})^{2})^{5} \cdot 3^{2}=3^{3\cdot 2\cdot 5 }\cdot 3^{2}=3^{30}\cdot 3^{2} =3^{30+2}=\huge\boxed{ 3^{32}}[/tex]
[tex]h)~~\left( \dfrac{9^{4}}{27} \right)^{2}=\left( \dfrac{(3^{2})^{4}}{3^{3}} \right)^{2}=\left( \dfrac{3^{2\cdot 4}}{3^{3}} \right)^{2}=\left( \dfrac{3^{8}}{3^{3}} \right)^{2}=(3^{8-3})^{2}=(3^{5})^{2}=3^{5\cdot 2} =\huge\boxed{3^{10}}[/tex]
-
Autor:
hailieeze8
-
Oceń odpowiedź:
9


