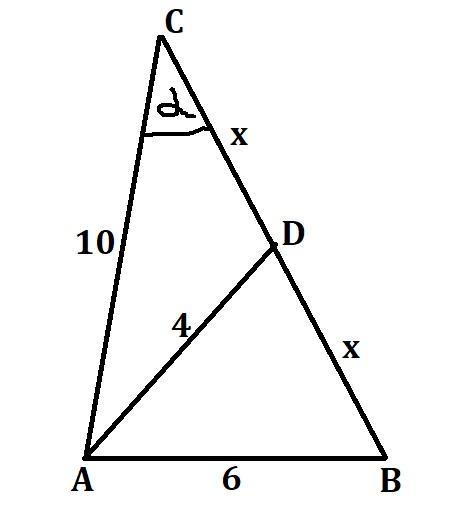
W trójkącie ABC środkowa AD ma długość 4, a boki AB i AC odpowiednio 6 i 10. Oblicz pole tego trójkąta. Czy ktoś coś na to poradzi?

Odpowiedź:

[tex]P_\triangle=24[/tex]

Szczegółowe wyjaśnienie:

Skorzystamy dwukrotnie z tw. cosinusów w trójkątach ABC i ADC.

[tex]\left \{ {{6^2=10^2+(2x)^2-2*10*2x*\cos\alpha} \atop {4^2=10^2+x^2-2*10*x*\cos\alpha}} \right. \\\left \{ {{36=100+4x^2-40x\cos\alpha} \atop {16=100+x^2-20x\cos\alpha}} \right. \\\left \{ {{40x\cos\alpha=4x^2+64\ |:40x} \atop {x^2-20x\cos\alpha+84=0}} \right. \\\left \{ {{\cos\alpha=\frac{4x^2+64}{40x}} \atop {x^2-20x\cos\alpha+84=0}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x^2-20x*\frac{x^2+16}{10x}+84=0}} \right.[/tex]

[tex]\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x^2-2*(x^2+16)+84=0}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x^2-2x^2-32+84=0}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {-x^2=-52}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x^2=52}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x=\sqrt{52}}} \right. \\\left \{ {{\cos\alpha=\frac{x^2+16}{10x}} \atop {x=2\sqrt{13}}} \right.[/tex]

[tex]\left \{ {{\cos\alpha=\frac{(2\sqrt{13})^2+16}{10*2\sqrt{13}}} \atop {x=2\sqrt{13}}} \right.\\\left \{ {{\cos\alpha=\frac{68}{20\sqrt{13}}} \atop {x=2\sqrt{13}}} \right.\\\left \{ {{\cos\alpha=\frac{17}{5\sqrt{13}}} \atop {x=2\sqrt{13}}} \right.[/tex]

Aby policzyć pole trójkąta, policzmy sinus kąta alfa.

[tex]\sin^2\alpha+\cos^2\alpha=1\\\sin^2\alpha+(\frac{17}{5\sqrt{13}})^2=1\\\sin^2\alpha+\frac{289}{325}}=1\\\sin^2\alpha=\frac{36}{325}}\\\sin\alpha=\sqrt{\frac{36}{325}}\vee\sin\alpha=-\sqrt{\frac{36}{325}}\\\sin\alpha=\frac{6}{5\sqrt{13}}}\vee\sin\alpha=-\frac{6}{5\sqrt{13}}}[/tex]

Sinus jest dodatni, niezależnie od tego, czy kąt alfa jest ostry czy rozwarty, więc

[tex]\sin\alpha=\frac{6}{5\sqrt{13}}}[/tex]

Policzmy pole trójkąta.

[tex]P_\triangle=\frac{1}{2}*|AC|*|BC|*\sin\alpha\\P_\triangle=\frac{1}{2}*10*4\sqrt{13}*\frac{6}{5\sqrt{13}}=20\sqrt{13}*\frac{6}{5\sqrt{13}}=\frac{120\sqrt{13}}{5\sqrt{13}}=24[/tex]